COGNITIVE SEMANTIC VISUALIZATION

ARTISTIC p-NUMBER GALLERY COGNITIVE SEMANTIC VISUALIZATION OF THE p-NUMBER . . . by

ALEXANDER & ANTON ZENKINS

Computing Center of the Russian Academy of Sciences
International Union of Artists

Incarnate faces of p (from 0 to 9)

The background itself of this site represents the same p-Number visualized according to the rule: all odd digits are gray and all even digits are yellow.
Further we shall visualize the distribution of different digits in the decimal representation of the p -Number. Consider, for example, the digit "0". 123456789*123456789*123456789*123456789*123456789* 31415926535897932384626433832795028841971693993751 05820974944592307816406286208998628034825342117067 98214808651328230664709384460955058223172535940812 84811174502841027019385211055596446229489549303819

644288109756659334461284756482337867831652........
(C) AZ

The Pythogram is being read (being counted) from left to right, and from top to bottom.

SOME DEFINITIONS AND REMARKS.

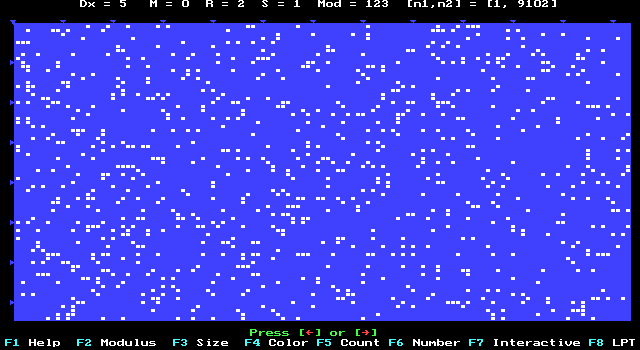
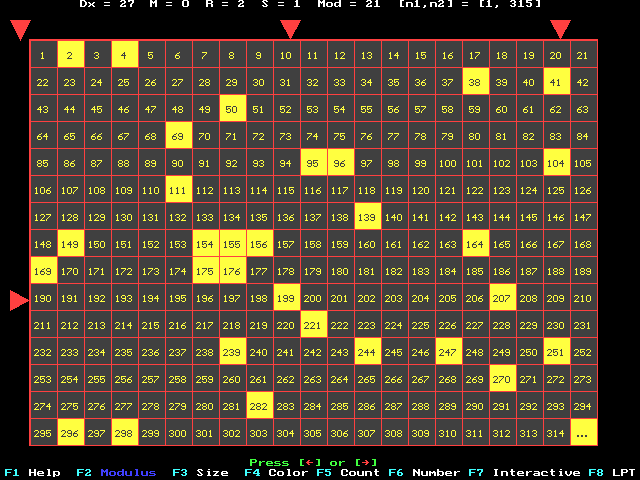
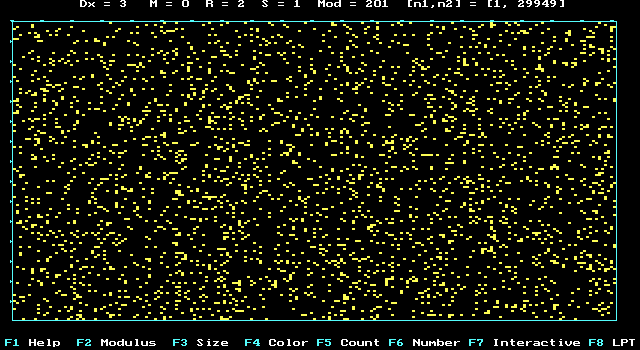
1. The Pythogram MODULUS is the quantity of numbers in any line.
2. The triangles over the first line and the left of the first column are marks of tens of columns and strings correspondingly
3. The Pythogram modulus (shown as "Mod = "),
    the size of squares (shown as "Dx = "),
    and the colors are chosen arbitrary.
4. Consecutively changing the modulus, we produce a unique computer p-film on the dynamics properties of the transcendency notion.
5. We have a version of the computer films for p , e, and any irrational numbers. But this is only part of CCG-System PYTHAGORAS (Dialogue System for Number Theory). This system can represent any kind of Number Theory additive problems and produce computer mathematical CCG-films for NT-experts as well as for a wide circle of scientists.
6. We have some p-projects :
    a) a video-film "Visual Comparison of the Cognitive Dynamical Color-Musical Images (Pythograms) of the TRANSCENDENT, ALGEBRAIC, and IRRATIONAL Numbers";
    b) color-musical, mental-aesthetic p-THERAPY of a soul;
    c) and others.
7. Unfortunately we do not have a possibility to complete the project here, so if you have been interested in a realization of such the project for science, art, and education we are ready for a collaboration. Please, e-mail us: alexzen@com2com.ru

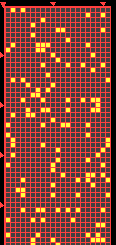
Let us consider now some admissible transformations of the pythograms.

For example, we can change the size of squares like this:

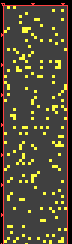Now we can change the modulus:

Or so:

   p-NUMBER GALLERY (SOME FRAGMENTS OF THE COMPUTER MATHEMATICAL FILM) "IF YOU SCRUTINIZE INTO THE ABYSS NARROWLY AND FOR A LONG TIME, THEN THE ABYSS ITSELF BEGINS TO PEER AT YOU . . ." ""ЕСЛИ ПРИСТАЛЬНО И ДОЛГО ВСМАТРИВАЕШЬСЯ В БЕЗДНУ, ТО БЕЗДНА САМА НАЧИНАЕТ СМОТРЕТЬ НА ТЕБЯ . . ." (Russian translation)

IMPORTANT REMARK:

now we recommend to change the screen distance
slowly back and forth from 3'' to 100''
to get the best cognitive effect. !!!

"0"- digit in p-Number:
123456789*123456789*123456789*123456789*123456789*
31415926535897932384626433832795028841971693993751
05820974944592307816406286208998628034825342117067
98214808651328230664709384460955058223172535940812
84811174502841027019385211055596446229489549303819
644288109756659334461284756482337867831652........
© AZ

"1"-digit in p-Number:
123456789*123456789*123456789*123456789*123456789*
31415926535897932384626433832795028841971693993751
05820974944592307816406286208998628034825342117067
98214808651328230664709384460955058223172535940812
84811174502841027019385211055596446229489549303819
644288109756659334461284756482337867831652........

© AZ

"2"- digit in p-Number:
123456789*123456789*123456789*123456789*123456789*
31415926535897932384626433832795028841971693993751
05820974944592307816406286208998628034825342117067
98214808651328230664709384460955058223172535940812
84811174502841027019385211055596446229489549303819
644288109756659334461284756482337867831652........
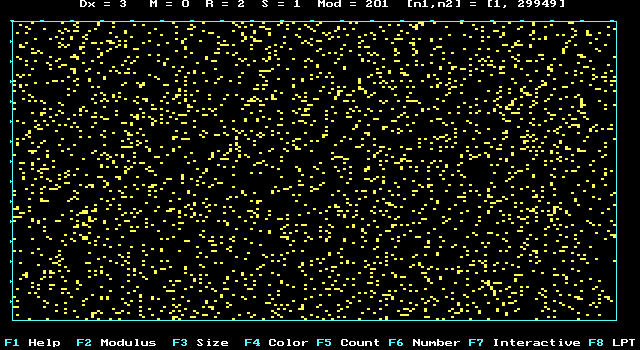© AZ

"3"- digit in p-Number:
123456789*123456789*123456789*123456789*123456789*
31415926535897932384626433832795028841971693993751
05820974944592307816406286208998628034825342117067
98214808651328230664709384460955058223172535940812
84811174502841027019385211055596446229489549303819
644288109756659334461284756482337867831652........
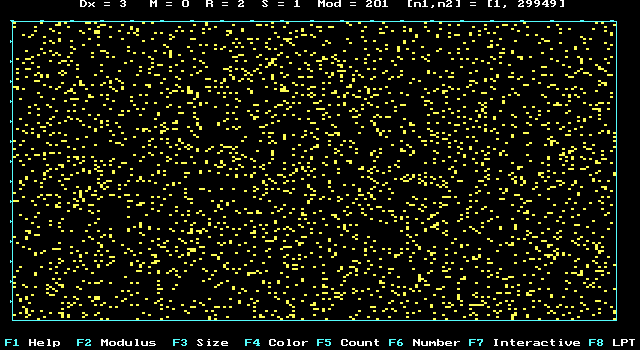© AZ

"4"- digit in p-Number:
123456789*123456789*123456789*123456789*123456789*
31415926535897932384626433832795028841971693993751
05820974944592307816406286208998628034825342117067
98214808651328230664709384460955058223172535940812
84811174502841027019385211055596446229489549303819
644288109756659334461284756482337867831652........
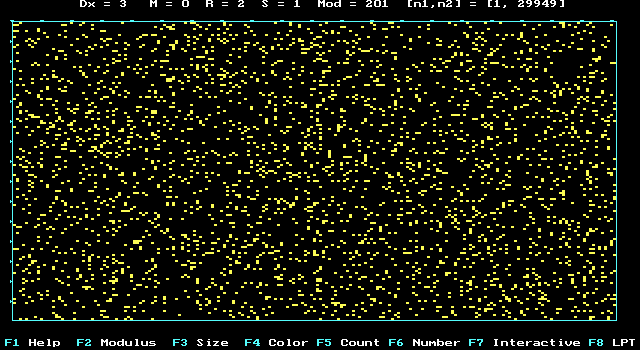© AZ

"5"- digit in p-Number:
123456789*123456789*123456789*123456789*123456789*
31415926535897932384626433832795028841971693993751
05820974944592307816406286208998628034825342117067
98214808651328230664709384460955058223172535940812
84811174502841027019385211055596446229489549303819
644288109756659334461284756482337867831652........
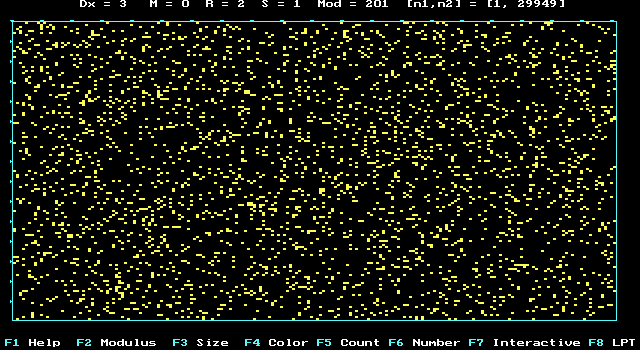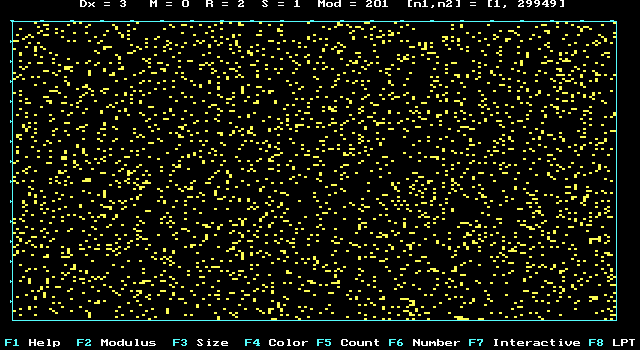
© AZ

"6"- digit in p-Number:
123456789*123456789*123456789*123456789*123456789*
31415926535897932384626433832795028841971693993751
05820974944592307816406286208998628034825342117067
98214808651328230664709384460955058223172535940812
84811174502841027019385211055596446229489549303819
644288109756659334461284756482337867831652........
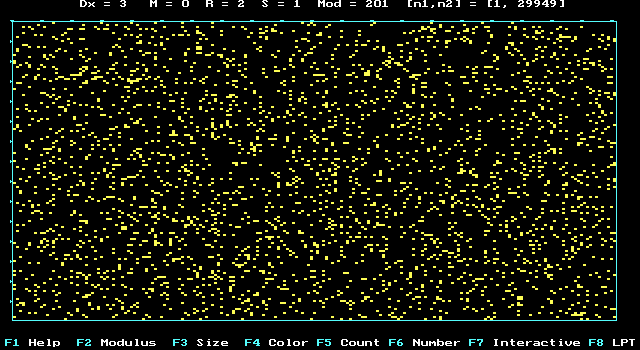
© AZ

"7"- digit in p-Number:
123456789*123456789*123456789*123456789*123456789*
31415926535897932384626433832795028841971693993751
05820974944592307816406286208998628034825342117067
98214808651328230664709384460955058223172535940812
84811174502841027019385211055596446229489549303819
644288109756659334461284756482337867831652........
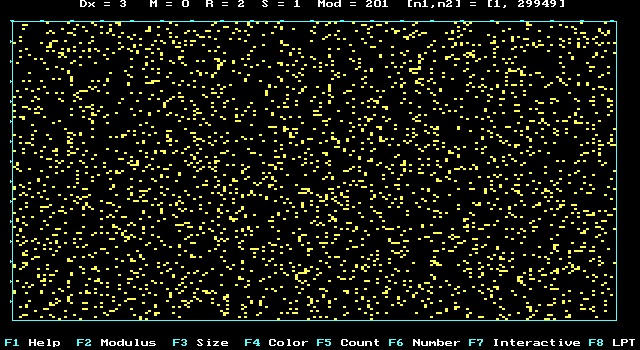
© AZ

"8"- digit in p-Number:
123456789*123456789*123456789*123456789*123456789*
31415926535897932384626433832795028841971693993751
05820974944592307816406286208998628034825342117067
98214808651328230664709384460955058223172535940812
84811174502841027019385211055596446229489549303819
644288109756659334461284756482337867831652........
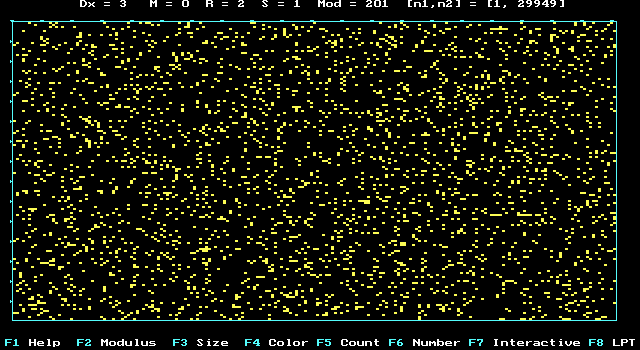
© AZ

"9"- digit in p-Number:
123456789*123456789*123456789*123456789*123456789*
31415926535897932384626433832795028841971693993751
05820974944592307816406286208998628034825342117067
98214808651328230664709384460955058223172535940812
84811174502841027019385211055596446229489549303819
644288109756659334461284756482337867831652........

© AZ

Some Color-Musical Mathematical Fantasies as to the Irrational Numbers ...
p -Number, an arbitrary colors. Dx=19.

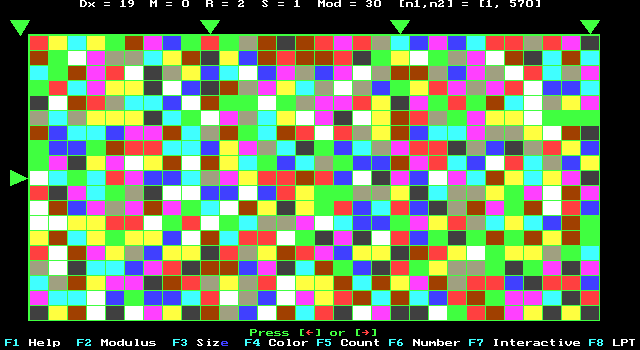(C)AZ

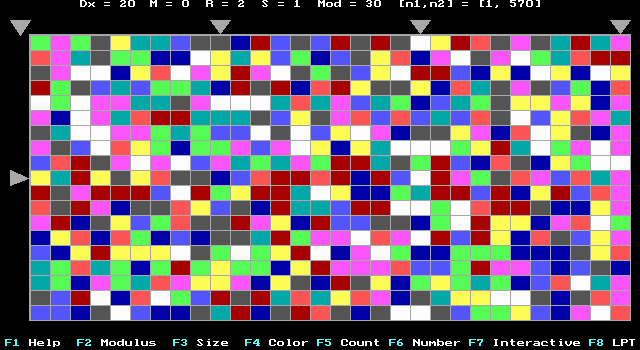
p -Number, an arbitrary colors. Dx=28. (a la Mandrian)

(C)AZ

p -Number, an arbitrary colors. Dx=2.
(C)AZ

p-Number: even (red)/odd(blue)
(C)AZ
p-Number: even (red)/odd(yellow)
(C)AZ

Main difference of our CCG-approach to the visualization of the p-Number from the an artistic approach:
The CCG-approach preserves the mathematical structure of the p-Number, and the corresponding pythograms contain ALL information about mathematical properties of the p-Number. So, some new, today unknown properties of that p-Number can be re-constructed, discovered by means of the investigation of VISUAL features of corresponding CCG-pythograms of the p-Number.
p-Number: even (yellow)/odd(gray)
(c) AZ

GOLDEN SECTION
GoldSection: an arbitrary colors (for every digit - its individual color.) 123456789*123456789*123456789*123456789*123456789*
16180339887498948482045868343656381177203091798..

(C)AZ

. (C)AZ

Golden Section: even (YELLOW)/odd(RED)

Magma

(C)AZ
Golden Section: even (blue)/odd(red)

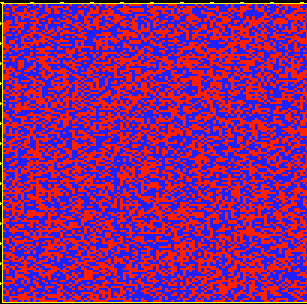Mosaic

COMMON PARTHS OF "p"-, "e"-, "Golden Section"-, and srt2- Numbers.

"p" x "e" = for any i=1: if p[i] = e[i] then BLACK else WHITE
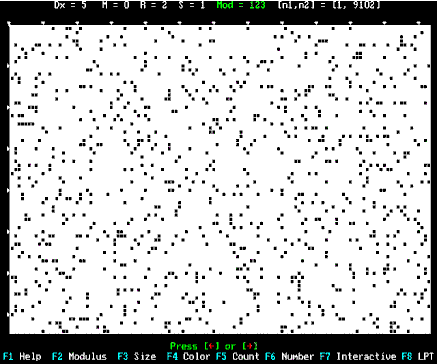(C)AZ

"p" x "GS" = for any i=1: if p[i] = GS[i] then BLACK else WHITE
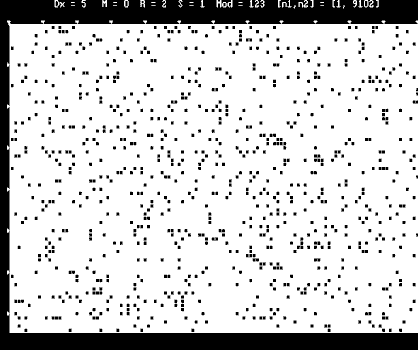(c)AZ

"e" x "GS" = for any i=1: if e[i] = GS[i] then BLACK else WHITE

(c)AZ


"p" x "e" x "GS" = for any i=1: if p[i] = e[i] = GS[i] then BLACK else WHITE

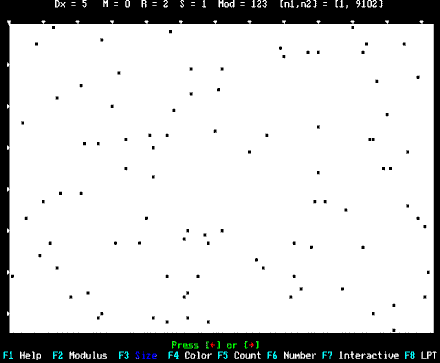
"p" x "sq2" = for any i > 1: if p[i] = sq2[i] then WHITE else BLUE

Magic PiWorld Gallery pictures by JVS & HLY http://www.jvshly.de/ That really is fine !

Contact: alexzen@com2com.ru back to CCG homepage